
Date: July 25, 2018
Author: Paul Cockshott
I have been producing a series of videos explaining materialism. Most of these have been accepted without controversy, but predictably enough, the last one, which was on quantum phenomena and materialism raised a number of critical comments.
The gist of my argument had been that the dominant Copenhagen interpretation of QM simply recapitulated the instrumentalist prejudices of the physicists of the early 20th century. The interpretation that, for instance, an electron or photon has no position until it is measured, merely restates the founding assumption of instrumentalism – that science is about relations between subjective sense data perceived by the scientist. This was extended to say that it is about relations between instrument readings that the scientist perceives.
Applied to QM we get notions to the effect that when one performs a two-slit experiment, the particles have no position until they are observed. That immediately poses the question as to what counts as an observation. The intuition from the philosophy and from the terminology ‘observer’ is that it human observers that count. But in actual physics experiments, the ‘observations’ are performed by machines or by things like photographic films. When a photon hits a CCD detector and is registered, does that count as an observation?
All of the experiments which are taken to justify the Copenhagen interpretation actually rely on ‘observations’ or recordings by such inanimate devices, so at this point there is nothing special about QM that relies on human observers. If we were to say that a CCD camera only really recorded something when a person looks at the result on a screen, then we are back in the sort of Berkeley type subjectivism where things cease to exist when we no longer look at them. This is absurd and no sort of foundation for scientific research. If, instead, we assume that it is interaction with macroscopic objects that ‘collapses the wave function’ as Copenhagenists put it, then one has two consequential problems:
- How big does something have to be to count as macroscopic? There appears to be no objectively determined scale at which something becomes macroscopic so I will not pursue this objection for now.
- How do you know that the photon had no position prior to it being detected? All that you definitely know from the experiment is that a particle was detected in a specific position, what is the basis for assuming that it did not have definite positions all along its path?
It is in the end just a philosophic assumption since there has existed for years a developed theory of quantum motion[2,1] under which particles do follow definite paths. This theory, known either as pilot wave theory or Bohm theory has been a contender with the Copenhagen theory since the 1950s. So when people say that the particle has no position until detected, they are either deliberately or inadvertently choosing to ignore the Bohm mechanics theory.
Delayed Eraser
There are, however, certain experiments, termed delay eraser experiments, which are taken to be hard to explain by anything other than the Copenhagen account. In this post, I will explain how this view is mistaken, and that these experiments provide no specific support for the Copenhagen view. I will do this by first examining the basic optical setup used in the delayed eraser experiments (Figure 1), then present several different uses of such an optical path, including that used in the delayed eraser test.
| start | slit | end |
| 0 | A | 1 |
| 0 | A | 2 |
| 0 | A | 3 |
| 0 | B | 1 |
| 0 | B | 2 |
| 0 | B | 4 |
| 1 | A | 0 |
| 2 | A | 0 |
| 3 | A | 0 |
| 1 | B | 0 |
| 2 | B | 0 |
| 4 | B | 0 |
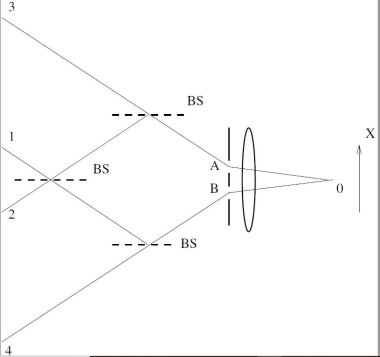
Figure 1 The basic optical path used in all the following diagrams. It is important to note that light can propagate along such optical paths in either direction. Possible paths tabulated below. Note the position 0 is intended to be scannable in the direction labeled X. Light reaching 0 through the slits or passing from 0 to the slits will follow a diffracted path.
Look at Figure 1. The lines indicate possible straight line light paths. There are 4 beam splitters, for example, half-silvered mirrors, which will reflect half of the light incident on them passing through the other half. There are 5 numbered positions, 0,1,2,3,4 and a set of possible light paths through the apparatus that are tabulated to the right of the diagram. The important thing to grasp is that light originating in or terminating in positions 1 or 2 can pass through either slit A or slit B. On the other hand light coming from or going to position 3 can only pass through slit A and light going to or coming from position 4 can only pass through slit B.

Figure 2A setup with four sources S1..4 and one detector D0. Assume one source at a time is on. Light from sources S3,4 passes through only one slit, and thus the diffracted pattern detected by D0 as it scans the X plane will have no interference fringes. On the other hand light from either S1 or from S2will be caused by the beam splitters to pass through both slits, giving rise to interference patterns. The space-time diagram indicates that the time taken to reach the slits T1 is greater than the time to go from the slit to the detector.
Now consider the setup in Figure 2. Here light is emitted by the sources S1..4, and depending on which source is switched on, will either generate an interference pattern to be picked up by the detector (S1or S2on) or generate no interference pattern ( S3or S4on).
This will be the case in practice and the result would be predicted either by classical wave optics or by a quantum theory such as the Copenhagen or the Bohm mechanics. There is nothing special about this.

Figure 3 The reversed quantum eraser thought the experiment of the type described in [3]. The dotted path on the space-time diagram is there for comparison with Figure 2.
Next, consider Figure 3 which is the ideal type model for the delayed eraser experiment. The first thing to note is that the optical path is the same as in the previous figures. What is different is that the slits are now assumed to be filled with some doped glass such that when excited by a pumping laser, atoms in the glass are raised to an excited state from which they can decay by emitting a pair of entangled photons. By conservation of momentum, the photons have to move off in opposite directions. Detectors are placed at positions 0 to 4. Detector D0can be linked via coincidence counters with any one of the detectors D1..4. The prediction of those who designed the experiment is that if one links D0with either D2or D3, scanning D0in the X plane will result in interference patterns of coincident counts whereas linking with D3or D4 will show no such interference pattern.
Clearly, the experimental apparatus will have to introduce a delay element in the linkage between D0and the counter to allow for the fact that photons will arrive at D0first. Alternatively one could record, with high accuracy when pulses were generated by all of the detectors and perform a post correlation analysis using appropriate lags to filter out non-entangled photons.
In [3]it was reported that the prediction is correct, interference coincidence patterns are only detected when correlating D0with D1and D2.
The Copenhagen style interpretation of this result is that when clicks are detected from D3or D4we know which path the photons took. In the case of D3they originate in slit A and in the case of D4 they originate in slit B. It is this knowledge of which slit the photons came from that prevents the interference pattern occurring in the temporally correlated clicks of D0. The apparently spooky effect is that knowledge acquired later seems to be influencing detections that occur earlier at D0 since T3>T2.
Discussion
The first point to note is that the laws of optics are time symmetric. That is to say, if one considers a 4 D space-time representation of an optical path through space and time, it remains a valid path under inversion of the time axis. This time symmetric property is true both of classical electromagnetism and of the pilot wave theory.
From this, it follows that there is nothing spooky about the post-detection at D1,2generating interference fringes in coincidence but not D3,4. Look at the space-time diagram of Figure 3. We have shown the time inverted path from the slit to S1 corresponding to the forward time path from the slit to D1. The pumping by the laser and entangled emission of photons is a means by which the space-time path in Figure 2 is rotated by 90 degrees to give the space-time path in Figure 3. The photon emitted towards D0is indistinguishable from one emitted by the corresponding source in Figure 2. In the previous case, it was easy to see that only emissions from S1,2could generate interference. In the previous experiment, this was done by selectively turning on individual sources to generate or not generate interference. In the so-called eraser experiment, the coincidence detector selects out the corresponding conjugate paths. The fact that in the first thought experiment the event on Si occurs before the event on D0whereas in the second experiment it occurs after, does not matter. All that the laws of optics have to do is set up symmetrical constraints in spacetime.
In Figure 3 we show the left-hand side of the space-time diagram mirror imaged. By symmetry, we should be able to set up a configuration in which both arms of the space-time diagram are mirror images. Consider Figure 4. Here we have replaced all the detectors in the previous figure with sources and the pumping laser with a detector tuned to the wavelength of the double energy photons. The sources would have to be set up in such a way that the source S0 and selectable source S1..4emit appropriately entangled photons with path lengths from the original source set up to be such that photons from left and right will arrive at slits A or B at the same time. If they arrive and coincide they will, by the same process as in dual photon confocal microscopy, stimulate the emission of double energy photons some of which will be picked up by the detector. By time symmetry of argument, when the optical paths are set up such that the entangled photons come in via S0and either S1or S2we will observe fluctuations in the rate of photon arrivals at the detector as So is scanned in direction X but no fluctuations if the entangled photons come in via S0and either S3or S4.
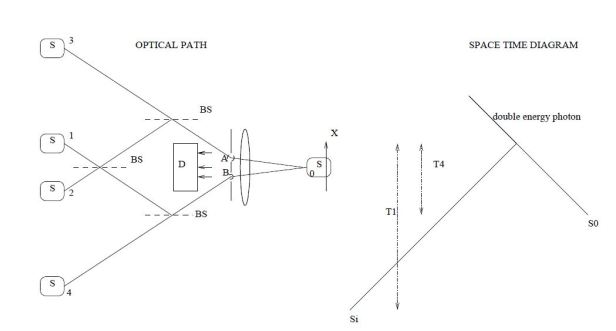
Figure 4 Fully time reversed advanced eraser experiment.
Conclusion
The phenomena observed in the delayed quantum eraser experiment are a necessary consequence of any time symmetric quantized laws of optics. There exist, as we have shown, a family of corresponding setups with partial or full time reversal of the paths. The delayed eraser can by symmetry be considered an advanced eraser. The experiments do not provide any specific support for the Copenhagen interpretation as against other time symmetric optics like the pilot wave theory.
Note that the argument I have given here is much influenced by the style of arguments presented in Huw Price’s book Times Arrow, Archimedes Point.
References
[1] Jean Bricmont. Making sense of quantum mechanics, volume 37. Springer, 2016.
[2] Peter R Holland. The quantum theory of motion: an account of the de Broglie-Bohm causal interpretation of quantum mechanics. Cambridge university press, 1995.
[3] Yoon-Ho Kim, Rong Yu, Sergei P Kulik, Yanhua Shih, and Marlan O Scully. Delayed “choice” quantum eraser. Physical Review Letters, 84(1):1, 2000.

